Factorial of 100?

The number is Factorial 100, which works in at 9.332622e+157. Find out the exact amount that the 100-factorial

The factororial 100 that is referred to by the numeral 100! is a huge number which represents the product of positive numbers that range from 1-100. Factorials are used extensively in maths, along with physics and computers, they are required for calculating permutations, combinations and probabilities. When numbers rise the value of factsorials increases exponentially and may even be 100! A complicated calculation. Understanding and calculating large factsorials, such as 100! requires effective algorithms and sophisticated computation tools, as standard calculators can’t cope with the complexity of these figures. This article is focused on the importance of factsorials and algorithms for computation and applications across a wide range of disciplines in science. Find out what’s the factorial for 100 numbers that are used in voice commands.

What is the Factorial of 100?

9.332622e+157
After calculation, the number for Factorial 100 turns out as equivalent to 9.332622e+157.

The factororial for 100 is described as
100
!
100! is a massive number:

100

!

93
,
326
,
215
,
443
,
944
,
152
,
681
,
699
,
238
,
856
,
266
,
700
,
490
,
715
,
968
,
264
,
381
,
621
,
468
,
592
,
963
,
895
,
217
,
599
,
993
,
229
,
915
,
608
,
941
,
463
,
976
,
156
,
518
,
286
,
253
,
697
,
920
,
827
,
223
,
758
,
251
,
185
,
210
,
916
,
864
,
000
,
000
,
000
,
000
,
000
,
000
,
000

100!=93,326,215,443,944,152,681,699,238,856,266,700,490,715,968,264,381,621,468,592,963,895,217,599,993,229,915,608,941,463,976,156,518,286,253,697,920,827,223,758,251,185,210,916,864,000,000,000,000,000,000,000

In simpler terms, it can be described by the name:

“Ninety-three quattuordecillion, three hundred twenty-six tredecillion, two hundred fifteen duodecillion, four hundred forty-three undecillion, nine hundred forty-four decillion, one hundred fifty-two nonillion, six hundred eighty-one octillion, six hundred ninety-nine septillion, two hundred thirty-eight sextillion, eight hundred fifty-six quintillion, two hundred sixty-six quadrillion, seven hundred trillion, four hundred ninety billion, seven hundred fifteen million, nine hundred sixty-eight thousand, two hundred sixty-four”

… and the list goes on.

Because it’s so big that we normally use it in a scientific terms like 100!9.3326×10 to generate to power

It is an useful way of expressing how important it is.

How can determine the exact value for Hundred(100)?

What is the answer what Factorial has 100?
100! is exactly: 93326215443944152681699238856266700490715968264381621468592963895217599993229915608941463976156518286253697920827223758251185210916864000000000000000000000000
Its approximate number is 9.3326215443944E+157.
A number that has zeros following within the 100th place has 24.
In reality the number of characters per factorial is 158.
The factorial value of 100 is determined through the definition of HTML0 in the following method:
100! = 100 * 99 * 98 * 97 * 96 … 3 * 2 * 1

What is Factorial?

The sum of positive numbers which are less than or equal to the positive integer given, in the form of that number and an exclamation point is known as the actorial math. At the end of the day, the number 7 is represented as 7! This is 7! which is 7. Factorial Zero is one. When looking at permutations, combinators, and factors that affect the coefficients that are found when analyzing binomial extensions factors are often encountered. The nonintegral value has been incorporated to factors. Factorials were first discovered in the writings of Jewish mystics, in the Talmudic Book Sefer Yetzirah and by Indian mathematicians in the classic texts from Jain literature. The process of factororialization is utilized in many mathematic fields, and is especially popular in combinatorics. This is the field that its main use is to determine how many unique combinations which is also known as permutations of an n-dimensible object, which are an infinitum number of factors! They are utilized in power series for the exponential function and other mathematical analyses, as well as being used in algebra, as well as in probability theory, number theory theory, and computer science.

The vast majority of the mathematical base to the factororial formula was devised during the 18th century and in the first half of the nineteenth century. Stirling’s approximation is similar to an effective factorial for large quantities exactly and shows that it operates faster that the exponential. Legendre’s formula could be used to calculate the quantity of factsorials zeros in the trail formulating exponents of prime numbers as factorization prime factsorials. The role of the factorial is to interpolate Daniel Bernoulli and Leonhard Euler to a unidirectional formula for complex numbers referred to as the Gamma function, with the exception of positive integers. Factorials are strikingly similar to other popular operations and sequences which include binomial coefficients, double factsorials falling factsorials, and subfactorials as well as primeorials. Functions that utilize the factorial principle are employed in computing calculators for scientific purposes and software libraries. They are frequently utilized as an example of different programming techniques for computers. Although computing huge factorials with the formula of recurrence or product could be inefficient, there are faster algorithms that are able to match the speed of fast multiplication procedures for numbers with the same number of digits to a certain amount of variables.

What is Factorial of 100- How to Calculate Factorial?

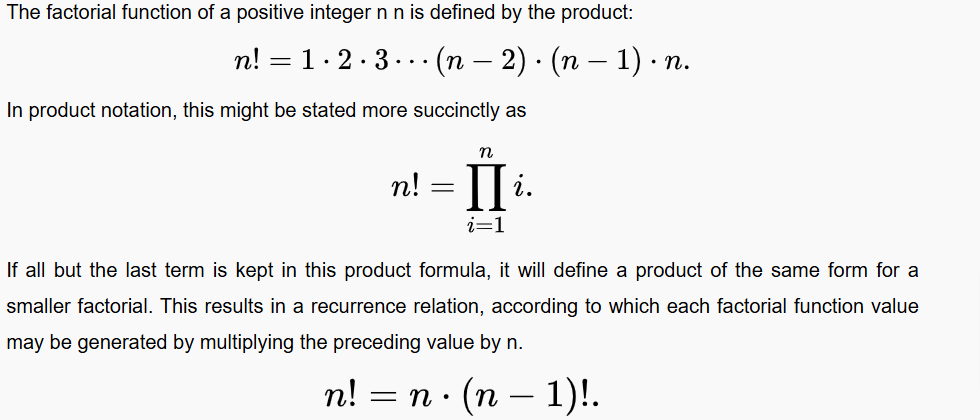
Factorial of 100- Applications of Factorial

The function of the factorial is initially used to count permutations: There are numerous ways to arrange different objects into the form of a sequence. Factorials are often employed in combinatorics formulas that are based on different orderings of items. The binomial coefficients (n k) are an excellent illustration. Calculate the k-element combinations (subsets that comprise K elements) from an array of an n-element set. They can be determined using factsorials. Factorials can be added on the stirling numbers of the one type and the combinations with the number of elements assembled into subsets which share the same number of cycles. Permutations or derangements that don’t remove any element from the original location can be used as an additional tool for combinatorial computation. The number of derangements within an element is equal to the nearest value to the integer n! (e).

The binomial theorem, which makes use of binomial coefficients to enhance the power of sums results in factsorials within math. They are also observed in the coefficients used to connect specific families of polynomials such as Newton’s identities with Symmetric polynomials. Factorials are the finite order of symmetric groups that explain their usage to calculate permutations in algebra. Factorials are component of the formula used by Faa di Bruno to link more complicated derivatives in the calculus. Factorials are often discovered in those denominators in the power series within math analysis, and more specifically in the series dealing with exponential formulas.